

Introduction

Nuclear fission takes place when a heavy atomic nucleus, such as uranium, breaks into two or more smaller pieces with
the release of some energy. During this process some of the mass of the original atom is converted into energy in
accordance with the equation E = mc
2
.
The idea that there might be a way to get at the energy locked up in an atom's mass took time to catch on. Einstein
himself thought it would never happen, and in an address given in England in 1933 the eminent atomic physicist and
discoverer of the nucleus of the atom, Ernest Rutherford, said:
"The energy produced by the breaking down of the atom is a very poor kind of thing.
Anyone who expects a source of power from the transformation of these atoms is
talking moonshine."
However, within 10 years the world's first nuclear reactor had been built and by the mid-1950s nuclear power stations
started supplying electrical power for industrial and domestic use.
Although the atoms of many different heavy elements undergo fission this page mainly concentrates on uranium, but with
plutonium, including how it is made, also discussed. We will start by explaining how fission takes place and then look at
some examples of its use.

Ernest Rutherford (1871-1937)
Discoverer of the nucleus of the atom

Isotopes and Half-life
From previous pages in this series we know an element is defined by the number of protons in its nucleus. For example,
carbon has 6 protons, but can have different numbers of neutrons also within its nucleus. Adding together the number of
protons and neutrons gives us the isotope of the element, such as carbon-11 or carbon-14. However, in all cases carbon
still has 6, and exactly 6, protons.
In the same way, there are different isotopes of uranium. By far the most common are uranium-238 (99.3%) and uranium-
235 (0.7%). In both cases they have 92 protons at their nucleus, and the bulk of each isotope is composed of neutrons.
Uranium-238 is a stable isotope, that is, it only rarely undergoes any form of radioactive decay. Uranium-235 is also stable
but undergoes radioactive decay a little more frequently.
All atoms decay, in other words, fall apart. Some of them take a very, very short time (a few billionths of a second) and
some a very, very long time (possibly 10
32
years for hydrogen). We can't say when an individual atom will decay, but we
can use statistical techniques to say how long it will be before a lot of atoms of a particular type decay to half of their
original amount. This is called the half-life of an element.
For example, carbon-14 has a half-life of 5730 years. That is, if we had a jar of carbon-14 and left it on a shelf for 5730
years half of it would have decayed into another element (nitrogen-14) and we would be left with only half the original
amount of carbon. Likewise, if we left it for another 5730 years, half of the carbon we had left would have decayed and no
longer be carbon, and so on:

Fission in Uranium-235
Uranium-235, while being less stable than uranium-238, is still quite a stable atom. If left by itself it has a half-life of 7.1 x
10
8
years. However, it was discovered that if an atom of uranium-235 is struck by a neutron (symbol n), the neutron
initially sticks to the atom to make uranium-236. This is a very unstable isotope and decays rapidly by splitting into
lighter atoms and particles. This is called induced fission. There are many different "channels" through which uranium-
235 can decay, i.e. there are many different particles that can result from the decay. We will look at the most common
one; that of uranium-235 splitting into barium, krypton and three neutrons.
First we fire a neutron (n) at the uranium-235 (U-235) atom so that it sticks to it. After a short while the uranium-235
splits into an atom of barium-141 (Ba-141), an atom of krypton-92 (Kr-92) and three neutrons. We can show this
schematically. Firstly, we see a neutron striking a uranium-235 atom to make a uranium-236 atom:
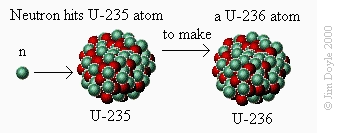
Secondly, the new uranium-236 atom rapidly decays into an atom of Ba-141 (barium), an atom of Kr-92 (krypton) and
three neutrons:
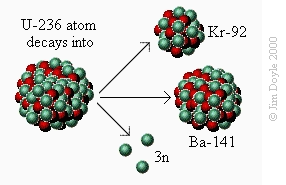
The resulting particles all have kinetic energy. This energy comes from converting a little of the mass of the original atom
into energy and can be measured using E = mc
2
. When this is done, the amount of energy typically released in the case
of U-235 is around 200 MeV (0.00000000003204 joules). That, it seems, is a very tiny amount of energy. However, it is
about a million times more energy than is released by the burning of one molecule of petrol (gas) in a car's engine. Put
another way, if you currently use a tank of petrol each week but could use the energy provided by one tank of uranium-
235 fission instead, you wouldn't need to re-fill your car for over 19,000 years.

Advertisement
Chain Reactions
We have seen that we can induce an atom of uranium-235 to undergo fission by bombarding it with neutrons. Does this
mean we have to keep bombarding a lot of U-235 with a lot of neutrons in order to get any useful energy out of it? No,
we let the uranium do it for us. In the previous section we saw that along with barium and krypton, three neutrons are
released during the fission process. These neutrons can hit further U-235 atoms and split them, releasing yet more
neutrons. This is called a chain reaction:
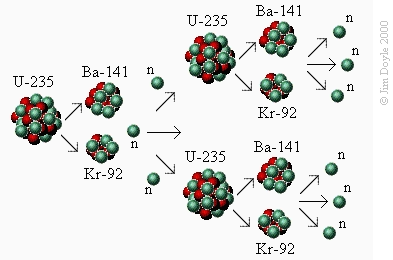
All we have to do is get enough U-235 in one place. If we do that we don't even need to supply the first neutron. Although
the half-life of U-235 is a very long time, if we get enough of the atoms together in one place the chances that any one of
them will undergo spontaneous fission is very, very high. This was first done at the end of 1942 in a disused squash court
under the University of Chicago's Stagg Field stadium. Graphite bricks containing U-235 were piled up in a box shape and a
further single brick was gradually inserted into the box. The insertion of the last brick was just enough to start a controlled
chain reaction in the U-235 as more and more neutrons were released as a result of nuclear fission. Looking back on this
event it is almost unbelievable that the experiment was allowed to go ahead. However, at the time no one was really sure of
the full health effects of radiation exposure and with World War II raging the safety of individuals was second to carrying out
what was seen as vitally important work. Not only had the scientists (lead by the brilliant Italian, later American, physicist
Enrico Fermi) created the world's first self-sustaining nuclear reactor, but had the last brick dropped into the "pile" they
would have been witness to the world's first nuclear meltdown.

Atomic Bombs
At the end of the previous section the word "meltdown" was used. A meltdown is when there is a runaway fission chain
reaction. More and more neutrons are released hitting more and more U-235 atoms, producing so much heat that
everything around the uranium melts. An atomic bomb can be thought of a very rapid meltdown.
The idea behind an atomic bomb is really very simple: bring lots of uranium-235 together very quickly so that it undergoes
very rapid fission. This can be done in a number of ways, for example by causing a conventional explosion around a lump
of high-grade (i.e. high density) U-235. This causes an "implosion", crushing the U-235 together to the point at which fission
very rapidly takes over and a huge explosion results as all the particles fly apart, together with a lot of heat and light that is
also released during the fission process.
Conventional bombs during the Second World War were filled with a derivative of the explosive TNT (trinitrotoluene). The
standard weight of the bombs dropped by heavy bombers was 1000 pounds (454 kg). The two atom bombs used in WWII
each had around the same explosive power as about 20 kilotons (20,000 tons, or 18,144 tonnes) of TNT. That is about the
same amount of explosive power as contained in about 40,000 conventional bombs. It's sobering to realise that the
enormous amount of explosive energy released when the first atomic bomb was dropped was produced by about the
amount of uranium-235 that could be held in a coffee mug.

Nuclear Power Stations
Nuclear power stations use another element that can undergo nuclear fission, that of plutonium (Pu), which is also used in
most modern atomic bombs. This element is slightly heavier than uranium, but doesn't occur naturally in anything like
sufficient quantities to be useful and so is made, or synthesized, from uranium together with other particles. The method is
surprisingly simple in that U-238 is bombarded with either neutrons until some stick and transmute (i.e. change) and so
form Pu-239, or the nuclei of heavy hydrogen (1 neutron and 1 proton) are used to bombard U-238 to form Pu-238. There
are intermediate stages of transmutation in each case, but what remains at the end is isotopes of plutonium, either Pu-239
or Pu-238, and with each nucleus containing 94 protons.
In this way the the vast bulk (99.3%) of mined uranium, i.e. U-238, can still be used to provide energy. The process of
turning uranium into plutonium is sometimes referred to as "breeding", and "breeder reactors" are used for this purpose.
A nuclear power station works in pretty much the same way as any other power station, only the energy source is different.
Generally, rods of fissionable material (plutonium) are pushed towards each other until a controlled amount of heat is
produced. This heat is used to produce steam, which is forced at high pressure through a set of turbine wheels. The turbine
wheels are connected to a generator and electricity is produced.
Producing electricity in this way is very cheap. In fact, during the 1950s it was even thought that when using nuclear fission
as the primary energy source electricity would be so cheap that it would be free to the end user because it wouldn't be
worth charging for it. However, while the actual conversion of radioactive energy (via E = mc
2
) into electrical energy is, on a
large scale, very cheap, other aspects of such an undertaking can be expensive. Nuclear power stations have problems
that other, conventional, power stations don't have, such as extra security costs and what to do with and how to store the
radioactive waste. On the other hand, conventional power stations have come under worldwide scrutiny in recent years
because of the levels of toxic and ozone destroying emissions, as well as with issues of global warming. Arguments about
which is the "best" source of electrical energy will no doubt continue for some time. In the meantime, as of 2021, nuclear
power is being used to generate around 10% of all electricity consumed around the globe, with many new reactors under
construction.
E = mc
2
Energy from the nucleus of an atom

Advertisement
Nuclear fission
Introduction


Nuclear fission takes place when a heavy atomic nucleus, such
as uranium, breaks into two or more smaller pieces with the
release of some energy. During this process some of the mass
of the original atom is converted into energy in accordance with
the equation E = mc
2
.
The idea that there might be a way to get at the energy locked
up in an atom's mass took time to catch on. Einstein himself
thought it would never happen, and in an address given in
England in 1933 the eminent atomic physicist and discoverer of
the nucleus of the atom, Ernest Rutherford, said:
"The energy produced by the breaking down of the
atom is a very poor kind of thing. Anyone who expects
a source of power from the transformation of these
atoms is talking moonshine."
However, within 10 years the world's first nuclear reactor had
been built and by the mid-1950s nuclear power stations started
supplying electrical power for industrial and domestic use.
Although the atoms of many different heavy elements undergo
fission this page mainly concentrates on uranium, but with
plutonium, including how it is made, also discussed. We will
start by explaining how fission takes place and then look at
some examples of its use.

Discoverer of the nucleus of the atom
Ernest Rutherford (1871-1937)

Isotopes and Half-life
From previous pages in this series we know an element is
defined by the number of protons in its nucleus. For example,
carbon has 6 protons, but can have different numbers of
neutrons also within its nucleus. Adding together the number of
protons and neutrons gives us the isotope of the element, such
as carbon-11 or carbon-14. However, in all cases carbon still
has 6, and exactly 6, protons.
In the same way, there are different isotopes of uranium. By far
the most common are uranium-238 (99.3%) and uranium-235
(0.7%). In both cases they have 92 protons at their nucleus,
and the bulk of each isotope is composed of neutrons.
Uranium-238 is a stable isotope, that is, it only rarely undergoes
any form of radioactive decay. Uranium-235 is also stable but
undergoes radioactive decay a little more frequently.
All atoms decay, in other words, fall apart. Some of them take a
very, very short time (a few billionths of a second) and some a
very, very long time (possibly 10
32
years for hydrogen). We
can't say when an individual atom will decay, but we can use
statistical techniques to say how long it will be before a lot of
atoms of a particular type decay to half of their original amount.
This is called the half-life of an element.
For example, carbon-14 has a half-life of 5730 years. That is, if
we had a jar of carbon-14 and left it on a shelf for 5730 years
half of it would have decayed into another element (nitrogen-14)
and we would be left with only half the original amount of
carbon. Likewise, if we left it for another 5730 years, half of the
carbon we had left would have decayed and no longer be
carbon, and so on:

Fission in Uranium-235
Uranium-235, while being less stable than uranium-238, is still
quite a stable atom. If left by itself it has a half-life of 7.1 x 10
8
years. However, it was discovered that if an atom of uranium-
235 is struck by a neutron (symbol n), the neutron initially sticks
to the atom to make uranium-236. This is a very unstable
isotope and decays rapidly by splitting into lighter atoms and
particles. This is called induced fission. There are many
different "channels" through which uranium-235 can decay, i.e.
there are many different particles that can result from the
decay. We will look at the most common one; that of uranium-
235 splitting into barium, krypton and three neutrons.
First we fire a neutron (n) at the uranium-235 (U-235) atom so
that it sticks to it. After a short while the uranium-235 splits into
an atom of barium-141 (Ba-141), an atom of krypton-92 (Kr-92)
and three neutrons. We can show this schematically. Firstly, we
see a neutron striking a uranium-235 atom to make a uranium-
236 atom:

Secondly, the new uranium-236 atom rapidly decays into an
atom of Ba-141 (barium), an atom of Kr-92 (krypton) and three
neutrons:

The resulting particles all have kinetic energy. This energy
comes from converting a little of the mass of the original atom
into energy and can be measured using E = mc
2
. When this is
done, the amount of energy typically released in the case of U-
235 is around 200 MeV (0.00000000003204 joules). That, it
seems, is a very tiny amount of energy. However, it is about a
million times more energy than is released by the burning of
one molecule of petrol (gas) in a car's engine. Put another way,
if you currently use a tank of petrol each week but could use the
energy provided by one tank of uranium-235 fission instead,
you wouldn't need to re-fill your car for over 19,000 years.

Advertisement
Chain Reactions
We have seen that we can induce an atom of uranium-235 to
undergo fission by bombarding it with neutrons. Does this mean
we have to keep bombarding a lot of U-235 with a lot of
neutrons in order to get any useful energy out of it? No, we let
the uranium do it for us. In the previous section we saw that
along with barium and krypton, three neutrons are released
during the fission process. These neutrons can hit further U-235
atoms and split them, releasing yet more neutrons. This is
called a chain reaction:

All we have to do is get enough U-235 in one place. If we do that
we don't even need to supply the first neutron. Although the half-
life of U-235 is a very long time, if we get enough of the atoms
together in one place the chances that any one of them will
undergo spontaneous fission is very, very high. This was first
done at the end of 1942 in a disused squash court under the
University of Chicago's Stagg Field stadium. Graphite bricks
containing U-235 were piled up in a box shape and a further
single brick was gradually inserted into the box. The insertion of
the last brick was just enough to start a controlled chain reaction
in the U-235 as more and more neutrons were released as a
result of nuclear fission.
Looking back on this event it is almost unbelievable that the
experiment was allowed to go ahead. However, at the time no
one was really sure of the full health effects of radiation
exposure and with World War II raging the safety of individuals
was second to carrying out what was seen as vitally important
work. Not only had the scientists (lead by the brilliant Italian,
later American, physicist Enrico Fermi) created the world's first
self-sustaining nuclear reactor, but had the last brick dropped
into the "pile" they would have been witness to the world's first
nuclear meltdown.

Atomic Bombs
At the end of the previous section the word "meltdown" was
used. A meltdown is when there is a runaway fission chain
reaction. More and more neutrons are released hitting more and
more U-235 atoms, producing so much heat that everything
around the uranium melts. An atomic bomb can be thought of a
very rapid meltdown.
The idea behind an atomic bomb is really very simple: bring lots
of uranium-235 together very quickly so that it undergoes very
rapid fission. This can be done in a number of ways, for
example by causing a conventional explosion around a lump of
high-grade (i.e. high density) U-235. This causes an "implosion",
crushing the U-235 together to the point at which fission very
rapidly takes over and a huge explosion results as all the
particles fly apart, together with a lot of heat and light that is also
released during the fission process.
Conventional bombs during the Second World War were filled
with a derivative of the explosive TNT (trinitrotoluene). The
standard weight of the bombs dropped by heavy bombers was
1000 pounds (454 kg). The two atom bombs used in WWII each
had around the same explosive power as about 20 kilotons
(20,000 tons, or 18,144 tonnes) of TNT. That is about the same
amount of explosive power as contained in about 40,000
conventional bombs. It's sobering to realise that the enormous
amount of explosive energy released when the first atomic
bomb was dropped was produced by about the amount of
uranium-235 that could be held in a coffee mug.

Nuclear power stations use another element that can undergo
nuclear fission, that of plutonium (Pu), which is also used in
most modern atomic bombs. This element is slightly heavier
than uranium, but doesn't occur naturally in anything like
sufficient quantities to be useful and so is made, or synthesized,
from uranium together with other particles. The method is
surprisingly simple in that U-238 is bombarded with either
neutrons until some stick and transmute (i.e. change) and so
form Pu-239, or the nuclei of heavy hydrogen (1 neutron and 1
proton) are used to bombard U-238 to form Pu-238. There are
intermediate stages of transmutation in each case, but what
remains at the end is isotopes of plutonium, either Pu-239 or
Pu-238, and with each nucleus containing 94 protons.
In this way the the vast bulk (99.3%) of mined uranium, i.e. U-
238, can still be used to provide energy. The process of turning
uranium into plutonium is sometimes referred to as "breeding",
and "breeder reactors" are used for this purpose.
A nuclear power station works in pretty much the same way as
any other power station, only the energy source is different.
Generally, rods of fissionable material (plutonium) are pushed
towards each other until a controlled amount of heat is
produced. This heat is used to produce steam, which is forced
at high pressure through a set of turbine wheels. The turbine
wheels are connected to a generator and electricity is produced.
Producing electricity in this way is very cheap. In fact, during the
1950s it was even thought that when using nuclear fission as
the primary energy source electricity would be so cheap that it
would be free to the end user because it wouldn't be worth
charging for it. However, while the actual conversion of
radioactive energy (via E = mc
2
) into electrical energy is, on a
large scale, very cheap, other aspects of such an undertaking
can be expensive. Nuclear power stations have problems that
other, conventional, power stations don't have, such as extra
security costs and what to do with and how to store the
radioactive waste. On the other hand, conventional power
stations have come under worldwide scrutiny in recent years
because of the levels of toxic and ozone destroying emissions,
as well as with issues of global warming. Arguments about
which is the "best" source of electrical energy will no doubt
continue for some time. In the meantime, as of 2021, nuclear
power is being used to generate around 10% of all electricity
consumed around the globe, with many new reactors under
construction.
Nuclear Power Stations
E = mc
2
Energy from the nucleus of an atom

Advertisement










